Navigation: Distance off a point method or double the angle
Each of our course pages has a nano-forum (called SeaTalks) attached so that students can comment on the content of that page. Recently a student asked a question on the nano-forum about the distance off/double the angle method and pointed out something which when we did the geometry analysis it turns up something quite revealing.
The distance off method uses basic geometry to determine the distance from an object without doing a fix on a chart. It is quite brilliant. First I’ll explain the concept then show you the failings of it.
The concept is basic triangle geometry.

Distance-off or double the angle method
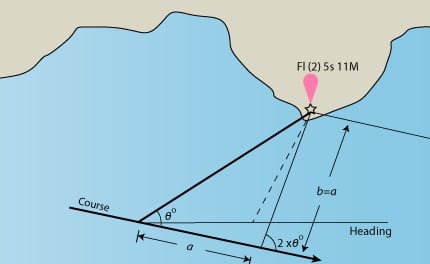
It says that when you have traveled a distance so that the angle to an object has doubled that the distance you traveled is the same as the distance from the object. All you have to know is your speed and the time you traveled. This comes from the fact that when the angle is doubled then the inside angle next to it is 180 – 2 x the angle. Thus since the sum of all angles in a triangle must = 180 then the unknown angle becomes the same. Thus it is an equilateral triangle and b must equal a.
So for example, say you are traveling at 5 knots and you spot a lighthouse ahead and to port @ time 1030. You measure the angle from your bow to the light house which you find to be 33 degrees. Over time you monitor the angle, when it reaches 66 degrees you note the time of 1105.
The time you traveled was: 35/60 = 0.583 hours
The distance then was speed x time = 5 knots x .583 hours = 2.92 nm
So simple! Right?
Here is the catch. What is your course? Do you know it? Not really, there is leeway (sideways pushing of your boat on the wind) and quite possibly current. The geometry equation fails when the heading (direction your boat is pointing) and the course over ground do not match. It is because you are measuring the angle from the bow to the object, which is not necessarily your course over ground. Often times these can be 10-20 degrees or more in a sailboat. Less so in a power boat where the speed is higher so current is less of a % and leeway is reduced.
All well if you know your course, but where did you get it from? GPS? Well then you definitely know where you are so you don’t need this method. 3 point fixes? Well then you definitely again have a chart and know where you are. So hmmmm what is one to do with this seemingly useless geometric wonderland that in practice does not work?
You could estimate your leeway and apply the correction to your heading and you could consult the tide current table to estimate the current effect of your heading. For example, say your leeway was 10 degrees to starboard in the example above (no current). Then the real angle from your course to the object would be 43 degrees. You should wait until the object then is at 86 degrees off your course which is 86-10 =76 degrees off your heading off the port bow.
Distance off with correction
We have this same discussion in our Coastal Navigation Course.
Take the NauticEd Coastal Navigation Course online NOW. Get real practical training and examples. Do it in your own time and take the tests as many times as you like – forever.





